Students of class 12 Mathematics should refer to MCQ Questions Class 12 Mathematics Linear Programming with answers provided here which is an important chapter in Class 12 Mathematics NCERT textbook. These MCQ for Class 12 Mathematics with Answers have been prepared based on the latest CBSE and NCERT syllabus and examination guidelines for Class 12 Mathematics. The following MCQs can help you to practice and get better marks in the upcoming class 12 Mathematics examination

Chapter 12 Linear Programming MCQ with Answers Class 12 Mathematics

MCQ Questions Class 12 Mathematics Linear Programming provided below have been prepared by expert teachers of grade 12. These objective questions with solutions are expected to come in the upcoming Standard 12 examinations. Learn the below provided MCQ questions to get better marks in examinations.

Question. The feasible region for an LPP is shown below:

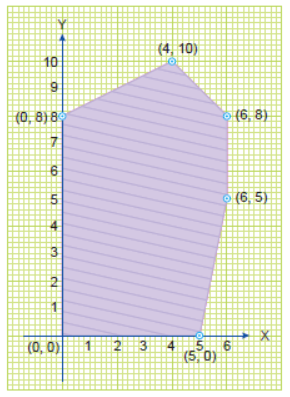
Let Z = 3x – 4y be the objective function. Minimum of Z occurs at
(a) (0, 0)
(b) (0, 8)
(c) (5, 0)
(d) (4, 10)

Question. The critical points of the function f (x)=2 sin² (X/6) + sin(x/3)-(x/3) whose coordinates satisfy the inequality x²-10<-19.5x, is
(a) -6π
(b) 6 π
(c) 9π/2
(d) – 4π 

Question. At what points in the interval [0 2π], does the function sin 2x attain its maximum value?
(a) x =π/4 and x=5π/4
(b) x =π/4 and x=3π/4
(c) x =-π/4 and x=-3/4
(d) None of these

Question.

Question. The number of critical points of f (x)= |x |(x-1) (x-2 ) (x-3 )  is
(a) 1
(b) 2
(c) 3
(d) 4

Question. The maximum and minimum values of x x + sin 2 on [0,2π] is 
(a) 2π and 0
(b) π and 1 /2
(c) π/2 and -1
(d) None of these

Question. It is given that at x = 1, the function x4-62x²+ ax +9  attains its maximum value, on the interval [ 0,2]. Find the value of a. 
(a) 30
(b) 40
(c) 75
(d) 120

Question. The maximum value of [x (x-1)+]^1/3,0≤ x ≤ 1 is
(a) (1/3)1/3
(b) 1/2
(c) 1
(d) zero

Question. All the points of local maxima and local minima of the function f (x)=-3/4 x⁴-8x³ – 45/2x² +105  are
(a) x= – 0 5, and x= – 3
(b) x=13, and x= – 2
(c) x= 0, -3, and x= – 2
(d) None of these

Question. The function f (x)=4x³-18x²+27x-7  18 27 7 3 2 has
(a) one local maxima
(b) one local minima
(c) one local maxima and two local minima
(d) neither maxima nor minima

Question. On the interval [0,1], the function x²⁵(1-x)⁷⁵  takes its maximum value at the point
(a) 0
(b) 1/4
(c) 1/2
(d) 1/3

Question. The value of ‘a’ for which the function f (x)= a sin x+1/3 sin3x has an extremum at x =π/3 is
(a) 1
(b) -1
(c) 2
(d) 0

Question. 

Question. The function f (x)=x +sin x has
(a) a minimum but no maximum
(b) a maximum but no minimum
(c) neither maximum nor minimum
(d) both maximum and minimum

Question. The function f (x)=x^-x,(x>0) attains a maximum value at x which is
(a) 2
(b) 3
(c) 1/e
(d) 1

Question. The function y= a (1- cos x) 1 is maximum when x is equal to
(a) π
(b) π / 2
(c) – π / 2
(d) – π / 6

Question. If a differential function f (x) has a relative minimum at x = 0, then the function Φ(x)=f(x)+ax +b has a relative minimum at x = 0 for
(a) all a and all b
(b) all b, if a = 0
(c) all b > 0
(d) all a > 0

Question. In (-4 ,4)  the function

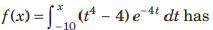
(a) no extrema
(b) one extremum
(c) two extrema
(d) four extrema

Question. The denominator of a fraction is greater than 16 of the square of numerator, then least value of fraction is
(a) -1 4/
(b) – 1 8/
(c) 1/12
(d) 1/16

Question. The function f (x)= ax +b/x, b, x>0 takes the least value at x equal to
(a) b
(b) a
(c) b
(d) b

Question. Which of the following statement is correct?
(a) Every LPP admits an optimal solution.
(b) Every LPP admits unique optimal solution.
(c) If a LPP gives two optimal solutions it has infinite number of solutions.
(d) None of these

Question. The solution set of the inequation 3x + 2y > 3 is
(a) half plane not containing the origin
(b) half plane containing the origin
(c) the point being on the line 3x + 2y = 3
(d) None of these

Question. In an LPP, if the objective function Z = ax + by has the same maximum value on two corner points of the feasible region, then the number of points of which Zmax occurs is
(a) 0
(b) 2
(c) finite
(d) infinite

Question. Corner points of the feasible region determined by the system of linear constraints are (0, 3),
(1, 1) and (3, 0). Let Z = px + qy, where p, q > 0. Condition on p and q so that the minimum of Z occurs at (3, 0) and (1, 1) is
(a) p = 2q
(b) p = q/2
(c) p = 3q
(d) p = q

Question. The optimal value of the objective function is attained at the points
(a) given by intersection of inequation with y-axis only.
(b) given by intersection of inequation with x-axis only.
(c) given by corner points of the feasible region.
(d) none of these

Question. Feasible region (shaded) for a LPP is shown in the given figure. Minimum of z = 4x + 3y occurs at the point

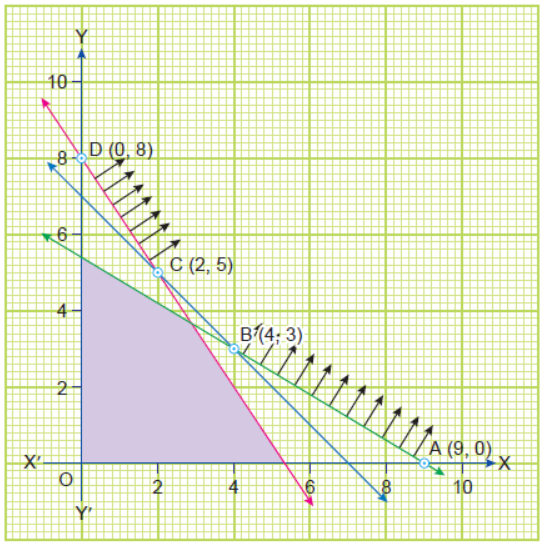
(a) (0, 8)
(b) (2, 5)
(c) (4, 3)
(d) (9, 0)

Question. If the constraints in a linear programming problem are changed
(a) solution is not defined
(b) the objective function has to be modified
(c) the problems is to be re-evaluated
(d) none of these

Question. The maximum value of Z = 4x + 3y subjected to the constraints 2x + 3y ≤ 18, x + y ≥ 10; x, y ≥ 0 is
(a) 36
(b) 40
(c) 20
(d) none of these

Question. The objective function Z = 4x + 3y can be maximised subjected to the constraints 3x + 4y ≤ 24,8x + 6y ≤ 48, x ≤ 5, y ≤ 6; x, y ≥ 0
(a) at only one point
(b) at two points only
(c) at an infinite number of points
(d) none of these

Question. The corner points of the feasible region of an LPP are (0, 0), (0, 8), (2, 7), (5, 4) and (6, 0). The maximum profit P = 3x + 2y occurs at the point ____________. 

Question. In a LPP, the linear function which has to be maximised or minimised is called a linear ____________ function. 

Question. In a LPP, the objective function is always ____________.     

Question. The common region determined by all the linear constraints of a LPP is called the ____________ region.    

Question. In a LPP, the inequalities or restrictions on the variables are called ____________.   

Question. If the feasible region for a LPP is ____________, then the optimal value of the objective function Z = ax + by may or may not exist.   

Question. The feasible region for an LPP is always a ____________ polygon.     

Question. The maximum value of Z = 6x + 16y satisfying the conditions x + y $ 2 , x $ 0, y $ 0 is ____________.     

Question. A corner point of a feasible region is a point in the region which is the ____________ of two boundary lines. 

We hope the above multiple choice questions for Class 12 Mathematics for Chapter 12 Linear Programming provided above with answers based on the latest syllabus and examination guidelines issued by CBSE, NCERT and KVS are really useful for you. Linear Programming is an important chapter in Class 12 as it provides very strong understanding about this topic. Students should go through the answers provided for the MCQs after they have themselves solved the questions. All MCQs have been provided with four options for the students to solve. These questions are really useful for benefit of class 12 students. Please go through these and let us know if you have any feedback in the comments section.