Please refer to Sample Paper Class 12 Physics Term 1 Set F with solutions provided below. We have provided CBSE Sample Papers for Class 12 Physics as per the latest paper pattern and examination guidelines for Standard 12 Physics issued by CBSE for the current academic year. The below provided Sample Guess paper will help you to practice and understand what type of questions can be expected in the Class 12 Physics exam.

CBSE Sample Paper Class 12 Physics for Term 1 Set F

SECTION – A

Question 1. What is the source of stellar energy?
Answer.Nuclear fusion.

Question 2. Distinguish between polar and non-polar dielectric.
OR
A hollow insulated conduction sphere is given a positive charge of 10 mC. What will be the electric field at the centre of the sphere if its radius is 2 metres ?
Answer. A dielectric whose molecules possess electric moment even when electric field is not applied is called polar dielectric. On the other hand, a dielectric whose molecules do not possess permanent dipole moment, is called non-polar dielectric.
OR
Field inside a conducting sphere = 0.

Question 3. State Huygens’ principle.
Answer. According to Huygens’ principle, each point on a wavefront is a source of secondary waves, which add up to give a wavefront at any later time.

Question 4. What is the value of time constant of a C-R circuit?
Answer. The time constant for resonance circuit, 𝜏 = CR

Question 5. A particle is dropped from a height H. Find the dependence of the de Broglie wavelength of the particle on height H.
OR
Figure shows a plot of 1/√V, where V is the accelerating potential,versus the de-Broglie wavelength ‘l’ in the case of two particles having same charge ‘q’ but different masses m₁ and m₂. Which line (A or B) represents a particle of large mass?

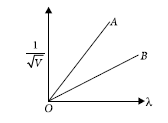
Answer.Velocity acquired by a particle while falling from a height H is

Question 6. Define ionisation energy. What is its value for a hydrogen atom?
Answer. Ionisation energy for an atom is defined as the energy required to remove an electron completely from the outermost shell of the atom.
The ionisation energy required to remove an electron from ground state of hydrogen atom is
E = E_∞ – E₁ = 0 – (–13.6) eV = 13.6 eV

Question 7. What is the electric flux linked with closed surface given in the figure?

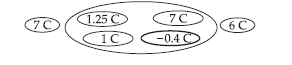
Answer.According to Gauss’s law

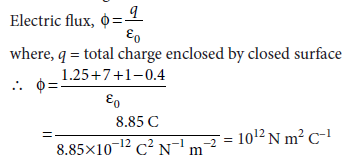
Question 8. Why is the penetrating power of gamma rays very large?
OR
What are the directions of electric and magnetic field vectors relative to each other and relative to the direction of propagation of electromagnetic waves?
Answer. g-rays are em waves of very large energy, so they passes through the matter with less number of collisions with atoms or molecules of matter, thereby penetrating more into it.

OR
In an electromagnetic wave E,B and direction of propagation are mutually perpendicular.

Question 9. Define 1 ampere current in terms of number of electrons flowing per second.
Answer.

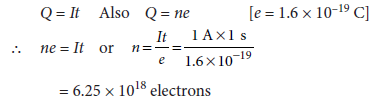
Question 10. An ac voltage is applied to a resistance R and an inductor L in series. If R and the inductive reactance are both equal to 3 W, calculate the phase difference between the applied voltage and the current in the circuit.
OR
An series L-C-R circuit is connected to a source of A.C. current. At resonance, what will be the phase difference between the applied voltage and the current in the circuit?
For question numbers 11, 12, 13 and 14, two statements are given-one labelled Assertion (A) and the other labelled Reason (R). Select the correct answer to these questions from the codes (a), (b), (c) and (d) as given sbelow.
(a) Both A and R are true and R is the correct explanation of A
(b) Both A and R are true but R is NOT the correct explanation of A
(c) A is true but R is false
(d) A is false and R is also false

Question 11. Assertion (A) : Gauss’s law shows diversion when inverse square law is not obeyed.
Reason (R) : Gauss’s law is a consequence of conservation of charges.

Question 12. Assertion (A) : A ray of light is incident from outside on a glass sphere surrounded by air. This ray may suffer total internal reflection at second interface.
Reason (R) : If a ray of light goes from denser to rarer medium, it bends away from the normal.

Question 13. Assertion (A) : The possibility of an electric bulb fusing is lower at the time of switching on and off.
Reason (R) : Inductive effects produce a decline in current at the time of switch-off and switch-on

Question 14. Assertion (A) : Accelerated charge radiate electromagnetic waves.
Reason (R) : As the wave propagate through the space, the oscillating electric and magnetic field regenerate each other.

SECTION – B

Question 15. Optical power (also referred to as dioptric power, refractive power, focusing power, or convergence power) is the degree to which a lens, mirror, or other optical system converges or diverges light. The SI unit for optical power is the inverse meter (m⁻¹), which is commonly called the dioptre. Converging lenses have positive optical power, while diverging lenses have negative power. When a lens is immersed in a refractive medium, its optical power and focal length change.

(i) What is the relation between the object distance u, image distance v and power P in a lens?

(a) uv/u − v
(b) u − v/u v
(c) uv
(d) u+v/u − v

(ii) The radius of curvature of each surface of a convex lens of refractive index 1.5 is 40 cm. Its power is
(a) 2.5 D
(b) 2 D
(c) 1.5 D
(d) 1 D

(iii) A convex lens of focal length 20 cm made of glass is immersed in a liquid of refractive index 2.3. Its power will be
(a) positive
(b) can be positive or Negative depending on the depth
(c) zero
(d) negative.

(iv) Focal length of a convex lens of refractive index 1.5 is 2 cm. Focal length of lens when immersed in a liquid of refractive index of 1.25 will be
(a) 5 cm
(b) 4 cm
(c) 2.5 cm
(d) 1.2 cm

(v) A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens.
The image is
(a) real, at 30 cm in front of the mirror
(b) real, at 30 cm behind the mirror
(c) real, at 10 cm in front of the mirror
(d) virtual, at 10 cm behind the mirror.

Question 16. A thermal power plant produced electric power of 600 kW at 4000 V, which is to be transported to a place 20 km away from the power plant for consumer’s usage. It can be transported either directly with a cable of large current carrying capacity or by using a combination of step‐up and step‐down transformers at the two ends. The drawback of the direct transmission is the large energy dissipation. In the method using transformers, the dissipation is much smaller. In this method a step‐up transformer is used at the plant side so that the current is reduced to a smaller value. At the consumers ‘end, a step‐down transformer is used to supply power to the consumers at the specified lower voltage. It is reasonable to assume that the power cable is purely resistive, and the transformers are ideal with power factor unity. All the currents and voltage mentioned are rms values.

In the method using the transformers assume that the ratio of the number of turns in the primary to that in the secondary in the step‐up transformer is 1 : 10 and the power to the consumers has to be supplied at 200 V.

(i) The voltage induced in the primary coil is
(a) 4000 V
(b) 40000 V
(c) 200 V
(d) 2000 V

(ii) If the direct transmission method with a cable of resistance 0.4 Ω km⁻¹ is used, the total current in the transmission line is
(a) 150 A
(b) 100 A
(c) 40 A
(d) 200 A

(iii) The power dissipation (in %) during transmission is
(a) 20
(b) 30
(c) 40
(d) 50

(iv) The ratio of the number of turns in the primary to that in the secondary in the step‐down transformer is
(a) 200 : 1
(b) 150 : 1
(c) 100 : 1
(d) 50 : 1

(v) The purpose of providing an iron core in a transformer is to
(a) provide support to windings
(b) reduce hysteresis loss
(c) decrease the reluctance of the magnetic path
(d) reduce eddy current losses

SECTION – C

Question 17. A charged conical conductor loses its charge earlier than a similarly charged sphere. Why?
Answer. In case of a conical conductor, the charge density is maximum at its pointed end. Due to this large density, the charge leaks away from this end. But in case of a similarly charged sphere, the charge density is uniform over its surface. Thus, the charged conical conductor loses its charge earlier than a similarly charged sphere.

Question 18. Name the electromagnetic waves used for studying crystal structure of solids. What is its frequency range?
Answer. X-rays, 3 × 10¹⁶ Hz to 3×10²⁰ Hz.

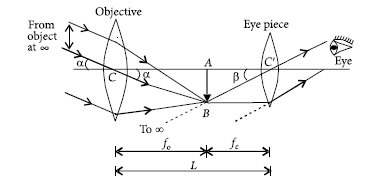
Question 19. Draw a ray diagram showing the image formation by an astronomical telescope when the final image is formed at infinity.
Answer.

Question 20. Consider the fusion reaction: ⁴He + ⁴He → ⁸Be
For the reaction, find (i) mass defect (ii) Q-value (iii) Is such a fusion energetically favourable?
Atomic mass of 8Be is 8.0053 u and that of 4He is 4.0026 u.

OR

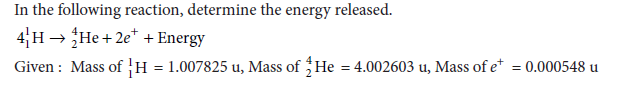
Answer.⁴He + ⁴He → ⁸Be + Q
(i) Dm = 2 × 4.0026 – 8.0053 = 8.0052 – 8.0053
= – 0.0001 amu
(ii) Q = (2m_He – m_Be) c² = (2 × 4.0026 – 8.0053) × 931 MeV
= –93.1 keV
(iii) Since Q is negative, the fusion is not energetically favourable.

OR
The given nuclear reaction is

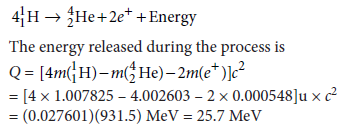
Question 21. If photons of frequency u are incident on the surfaces of metals A and B of threshold frequencies u/2 and u/3 respectively, find the ratio of the maximum kinetic energy of electrons emitted from A to that from B.
Answer.

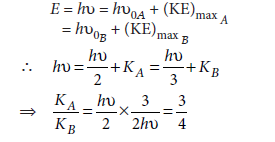
Question 22. A battery of emf 2 V is applied across the block of a semiconductor. The length of the block is 0.1 m and the area of cross-section is 1 × 10^–4 m². If the block is of intrinsic silicon at 300 K, find the electron and hole currents. [Take n_e = n_h = 1.5 × 10¹⁶/ m³; me = 0.135 m²V^–1s^–1; mn = 0.048 m²V^–1 s^–1]
Answer. For silicon:

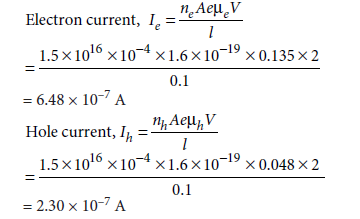
Question 23. What is the effective capacitance between points X and Y ?

Answer.The given circuit can be simplified as

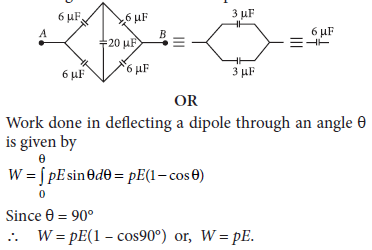
Question 24. What are eddy currents? Write any two applications of eddy currents.
OR
A bar magnet is moved in the direction indicated by the arrow between two coils PQ and CD. Predict the direction of the induced current in each coil.

Answer.When magnetic flux linked with a metallic conductor changes, then induced currents are set up in the conductor in the form of closed loops known as ‘Eddy currents’.
Two applications of eddy currents are
(i) electromagnetic braking in trains
(ii) induction furnace
OR

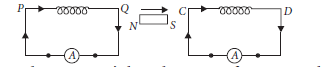
According to Lenz’s law, direction of current in loop PQ is from P to Q and in loop CD is from C to D.

Question 25. In the given circuit, find the current through the battery.

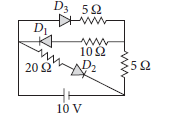
Answer. In the given circuit, diode D₁ is reverse biased, so it will not conduct. Diodes D₂ and D₃ are forward biased, so they will conduct. The corresponding equivalent circuit is as shown in the figure.
The equivalent resistance of the circuit is

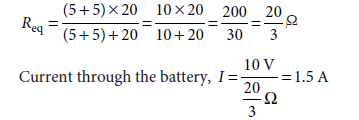
SECTION – D

Question 26. How does an oscillating charge produce electromagnetic wave? Explain. Draw a sketch showing the propagation of plane e.m. wave along the Z-direction, clearly depicting the directions of oscillating electric and magnetic field vectors.
Answer.  An oscillating or accelerated charge is supposed to be source of an electromagnetic wave. An oscillating charge produces an oscillating electric field in space which further produces an oscillating magnetic field which in turn is a source of electric field. These oscillating electric and magnetic field hence, keep on regenerating each other and an electromagnetic wave is produced.
The e.m. wave propagates along z-axis.

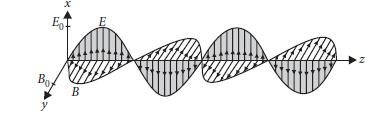
For an e.m. wave propagating in Z-direction, electric field is directed along X-axis and magnetic field is directed along Y-axis.

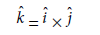
Question 27. A solenoid has a core of a material with relative permeability 400. The windings of the solenoid are insulated from the core and carry a current of 2 A. If the number of turns is 1000 per metre, calculate (i) H (ii) B (iii) the magnetising current, I_m.
OR
Where on the earth’s surface is the value of vertical component of the earth’s magnetic field zero? The horizontal component of the earth’s magnetic field at a given place is 0.4 × 10^–4 Wb m^–2 and angle of dip is 30°. Calculate the value of (i) vertical component, (ii) the total intensity of the earth’s magnetic field.
Answer.Here, μ_r = 400, I = 2 A, n = 1000 per metre
(i) H = nI= 1000 × 2 = 2 × 10³ A m^–1
(ii) B = μH = μ₀μ_r H = 4π × 10^–7 × 400 × (2 × 10³)
= 1.0 T
(iii) The magnetising current I_m is the additional current that needs to be passed through the windings of the solenoid in the absence of the core, which would produce a B value as in the presence of the core.
Thus, B = μ₀n(I + I_m)

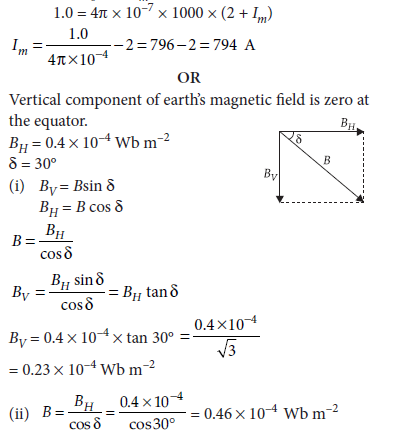
Question 28. A convex lens made of a material of refractive index μ₁ is kept in a medium of refractive index m2. Parallel rays of light are incident on the lens. Complete the path of rays of light emerging from the convex lens if
(a) μ₁ > μ₂
(b) μ₁ = μ₂
(c) μ₁ < μ₂
Answer.In case (a) μ₁ > μ₂, the lens behaves as convergent lens.
In case (b) μ₁ = μ₂, the lens behaves as a plane plate.
In case (c) μ₁ < μ₂, the lens behaves as a divergent lens.
The path of rays in all the three cases is shown in the figure.

Question 29. Mobilities of electrons and holes in a sample of intrinsic germanium at room temperature are 0.36 m² V^–1 s^–1 and 0.17 m² V^–1 s^–1. The electron and hole densities are each equal to 2.5 × 1019 m^–3. Determine the electrical conductivity of germanium.
OR
Write any two distinguishing features between conductors, semiconductors and insulators on the basis of energy band diagrams.
Answer.The electrical conductivity of germanium is

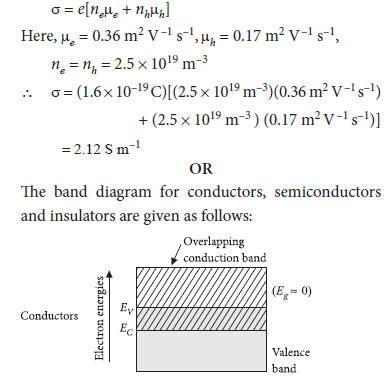
(i) In conductors, the valence band and conduction band tend to overlap (or nearly overlap) while in insulators they are separated by a large energy gap and in semiconductors they are separated by a small energy gap.
(ii) The conduction band of a conductor has a large number of electrons available for electrical conduction.
However, the conduction band of insulators is almost empty while that of the semiconductor has only a (very) small number of such electrons available for electrical conduction.

Question 30. An electric dipole is kept first to the left and then to the right of a negatively charged infinite plane sheet having a uniform surface charge density. The arrows p1 and p2 show the directions of its electric dipole moment in the two cases. (a) Identify for each case, whether the dipole is in stable or unstable equilibrium.
Justify each answer. 

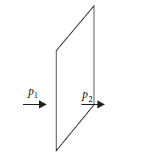
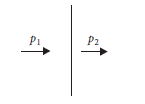
(b) Next, the dipole is kept in a similar way (as shown), near an infinitely long straight wire having uniform negative linear charge density. Will the dipole be in equilibrium at these two positions? Justify your answer. 

Answer.(a) p₁: stable equilibrium p₂: unstable equilibrium
The electric field, on either side, is directed towards the negatively charged sheet and its magnitude is independent of the distance of the field point from the sheet.
For position p_1, dipole moment and electric field are parallel, while for position p₂, they are antiparallel.
Hence, p₁ is in stable equilibrium and p₂ is in unstable equilibrium.
(b) The dipole will not be in equilibrium in any of the two positions.
The electric field due to an infinite straight charged wire is non- uniform (E ∝ 1/r).
Hence there will be a net non-zero force on the dipole in each case.

SECTION – E

Question 31. A gas of hydrogen like atoms can absorb radiations of 68 eV. Consequently, the atoms emit radiations of only three different wavelength. All the wavelengths are equal or smaller than that of the absorbed photon.
(i) Determine the initial state of the gas atoms.
(ii) Identify the gas atoms.
(iii) Find the minimum wavelength of the emitted radiations.
(iv) Find the ionization energy and the respective wavelength for the gas atoms.
OR
Write shortcomings of Rutherford’s atomic model. Explain how these were overcome by the postulates of Bohr’s atomic model.
Answer.

i.e. after excitation, atom jumps to second excited state.
Hence, nf = 3. So ni can be 1 or 2.
If ni = 1 then energy emitted is either equal to, greater than or less than the energy absorbed. Hence, the emitted wavelength is either equal to, less than or greater than the absorbed wavelength.

OR
Limitation of Rutherford’s model :
Rutherford’s atomic model is inconsistent with classical physics.
According to electromagnetic theory, an electron is a charged particle moving in the circular orbit around the nucleus and is accelerated, so it should emit radiation continuously and thereby loose energy. Due to this, radius of the electron would decrease continuously and also the atom should then produce continuous spectrum, and ultimately electron will fall into the nucleus and atom will collapse in 10^–8 s. But the atom is fairly stable and it emits line spectrum.

(ii) Rutherford’s model is not able to explain the spectrum of even most simplest H-spectrum.
Bohr’s postulates to resolve observed features of atomic spectrum :
(i) Quantum condition: Of all the possible circular orbits allowed by the classical theory, the electrons are permitted to circulate only in those orbits in which the angular momentum of an electron is an integral multiple of h/ 2πH, being Planck’s constant. Therefore, for any permitted orbit,

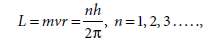
where n is called the principal quantum number, and this equation is called Bohr’s quantisation condition.
(ii) Stationary orbits: While resolving in the permissible orbits, an electron does not radiate energy.
These non-radiating orbits are called stationary orbits.
(iii) Frequency condition: An atom can emit or absorb radiation in the form of discrete energy photons only when an electron jumps from a higher to a lower orbit or from a lower to a higher orbit, respectively.
hu = E_i – E_f
where u is frequency of radiation emitted, Ei and Ef are the energies associated with stationary orbits of principal quantum number ni and nf respectively
(where n_i > n_f).

Question 32. A 2 μF capacitor, 100 W resistor and 8 H inductor are connected in series with an ac source
(i) What should be the frequency of the source such that current drawn in the circuit is maximum? What is this frequency called?
(ii) If the peak value of emf of the source is 200 V, find the maximum current.
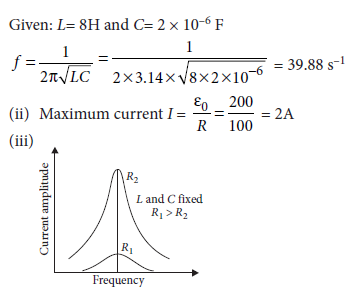
(iii) Draw a graph showing variation of amplitude of circuit current with changing frequency of applied voltage in a series LCR circuit for two different values of resistance R₁ and R₂ (R₁ > R₂).
(iv) Define the term ‘Sharpness of Resonance’. Under what condition, does a circuit become more selective?
OR
A device X is connected across an ac source of voltage V = V₀sinwt. The current through X is given as

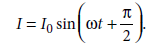
(a) Identify the device X and write the expression for its reactance.
(b) Draw graphs showing variation of voltage and current with time over one cycle of ac, for X.
(c) How does the reactance of the device X vary with frequency of the ac? Show this variation graphically.
(d) Draw the phasor diagram for the device X.
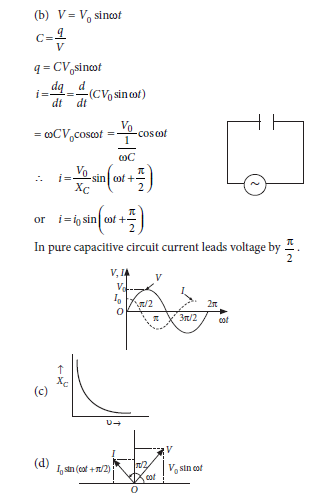
Answer.(i) For maximum current , the impedence should be minimum, i.e., XL= XC.
In such a condition the frequency is called as resonance frequency and is given by

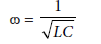
(iv) Sharpness of resonance : It is defined as the ratio of the voltage developed across the inductance (L) or capacitance (C) at resonance to the voltage developed across the resistance (R).

It may also be defined as the ratio of resonant angular frequency to the bandwidth of the circuit.
Q = ω_r/2Δω
Circuit become more selective if the resonance is more sharp, maximum current is more, the circuit is close to resonance for smaller range of (2Dw) of frequencies.
Thus, the tuning of the circuit will be good.
Figure shows the variation of im with w in a LCR series
circuit for two values of resistance R1 and R₂(R₁ > R₂),

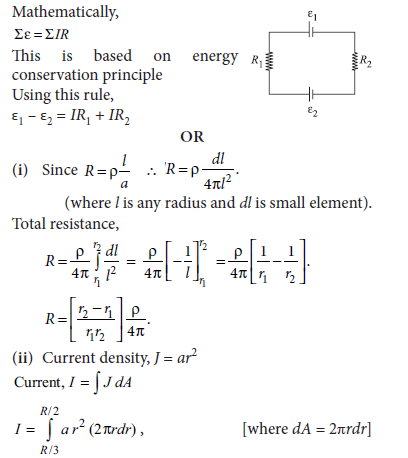
Question 33. State the two Kirchhoff’s laws. Explain briefly how these rules are justified.
OR
(i) Space between two concentric spheres of radii r₁ and r₂, such that r₁ < r₂, is filled with a material of resistivity r. Find the resistance between inner and outer surface of the material. 

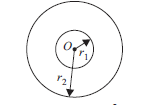
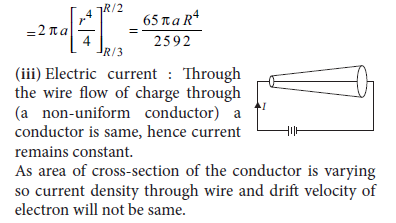
(ii) The current density varies with radial distance r as J = ar2, in a cylindrical wire of radius R. Determine the current passing through the wire between radial distance R/3 and R/2.
(iii) A wire whose cross-sectional area is increasing linearly from its one end to the other, is connected across a battery of V volts. Which of the following quantities remain constant in the wire?
(a) drift speed (b) current density (c) electric current (d) electric field Justify your answer.
Answer. Kirchhoff’s first rule : The algebraic sum of all the current passing through a junction of an electric circuit is zero.
Here, I₁, I₂, I₃, I₄ and I₅ are current in different branches
of a circuit which meet at a junction.
I₁ + I₂ – I₃ + I₄ – I₅ = 0

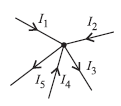
This rule is based on the principle of conservation of charge.
Kirchhoff’s second rule : The algebraic sum of the applied emf’s of an electrical circuit is equal to the algebraic sum of potential drops across the resistors of the loop.