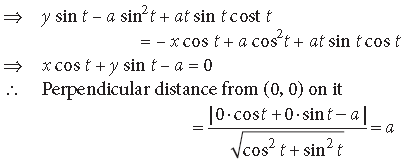Please refer to below Exam Question for Class 12 Mathematics Chapter 6 Application of Derivatives. These questions and answers have been prepared by expert Class 12 Mathematics teachers based on the latest NCERT Book for Class 12 Mathematics and examination guidelines issued by CBSE, NCERT, and KVS. We have provided Class 12 Mathematics exam questions for all chapters in your textbooks. You will be able to easily learn problems and solutions which are expected to come in the upcoming class tests and exams for standard 10th.

Chapter 6 Application of Derivatives Class 12 Mathematics Exam Question

All questions and answers provided below for Exam Question Class 12 Mathematics Chapter 6 Application of Derivatives are very important and should be revised daily.

Exam Question Class 12 Mathematics Chapter 6 Application of Derivatives

Very Short Answer Type Questions

Question. Show that the function f given by f(x) = x³ – 3x² + 4x, x ∈R is strictly increasing on R.
Answer. Here f(x) = x³ – 3x² + 4x
⇒ f’ (x) = 3x² – 6x + 4 = 3(x² – 2x) + 4
= 3(x² – 2x + 1) – 3 + 4
= 3(x – 1)² + 1 > 0 ∀ x ∈ R
⇒ f is strictly increasing on R.

Question. The amount of pollution content added in air in a city due to x-diesel vehicles is given by P(x) = 0.005x³ + 0.02x² + 30x. Find the marginal increase in pollution content when 3 diesel vehicles are added and write which value is indicated in the above question.
Answer. We have, P(x) = 0.005x³ + 0.02x² + 30x

Value indicated in the question is that the increase in pollution is due to the increase in use of diesel vehicles.

Question. The money to be spent for the welfare of the employees of a firm is proportional to the rate of change of its total revenue (marginal revenue). If the total revenue (in rupees) received from the sale of x units of a product is given by R(x) = 3x² + 36x + 5, find the marginal revenue, when x = 5, and write which value does the question indicate.
Answer. Total revenue is given by
R(x) = 3x² + 36x + 5

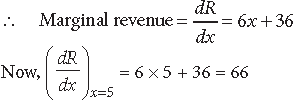
Value indicated in the question is that more amount of money is spent for the welfare of the employees with the increase in marginal revenue.

Short Answer Type Questions

Question. The side of an equilateral triangle is increasing at the rate of 2 cm/s. At what rate is its area increasing when the side of the triangle is 20 cm?
Answer. Let ‘a’ be the side of the equilateral triangle.
Then da/dt = 2cm/ sec
Let ‘A’ be the area of the equilateral triangle, then

Question. A ladder 5 m long is leaning against a wall. The bottom of the ladder is pulled along the ground, away from the wall, at the rate of 2 cm/s. How fast is its height on the wall decreasing when the foot of the ladder is 4 m away from the wall? 
Answer. Let foot of the ladder is at a distance of ‘x’ from the wall and height of the wall is ‘y’. Here, x² + y² = 52

Question. Sand is pouring from a pipe at the rate of 12 cm³/sec. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is 4 cm ?
Answer. Let at any instant of time t, the radius of the base of the cone be r, its height be h and the volume of cone be V, then

Question. The length x of a rectangle is decreasing at the rate of 5 cm/minute and width y is increasing at the rate of 4 cm/minute. When x = 8 cm and y = 6 cm, find the rate of change of (a) the perimeter, (b) the area of the rectangle.
Answer. Let at any instant of time t, the perimeter of the rectangle be P and the area be A

∴ Area of the rectangle is increasing at the rate of 2 cm²/min.

Question. Find the intervals in which the function f(x) = 3x⁴ – 4x³ – 12x² + 5 is
(a) strictly increasing
(b) strictly decreasing
Answer. We have, f(x) = 3x⁴ – 4x³ – 12x² + 5
f’ (x) = 12x³ – 12x² – 24x = 12x(x² – x – 2)
⇒ f’ (x) = 12x(x + 1)(x – 2)
Now, f’ (x) = 0
⇒ 12x(x + 1)(x – 2) = 0 ⇒ x = –1, x = 0 or x = 2
Hence these points divide the whole real line into
four disjoint open intervals namely (–∞, –1), (–1, 0), (0, 2) and (2, ∞)

(a) f(x) is strictly increasing in (–1, 0) U (2, ∞)
(b) f(x) is strictly decreasing in (–∞, –1) U (0, 2)

Question. Find the value(s) of x for which y = [x (x–2)]² is an increasing function.
Answer. Here, y = [x(x – 2)]² = x²(x – 2)²

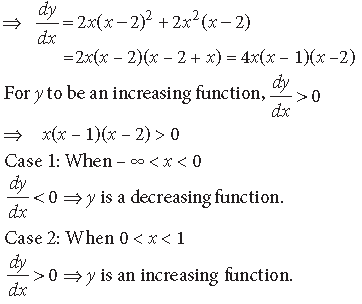
Question. Find the intervals in which the function

Answer.

Hence, the points divide the real line into disjoint intervals (–∞, –2), (–2, 1), (1, 3) and (3, ∞)

(a) f(x) is strictly increasing in (–2, 1) U (3, ∞)
(b) f(x) is strictly decreasing in (– ∞, –2) U (1, 3)

Question. Find the intervals in which the following function is (a) increasing (b) decreasing :
f(x) = 2x³ – 9x² + 12x – 15 
Answer. Here, f(x) = 2x³ – 9x² + 12x – 15
⇒ f’ (x) = 6x² – 18x + 12
= 6(x² – 3x + 2) = 6(x – 2) (x – 1)
(a) For f to be increasing,
f’ (x) > 0 ⇒ 6(x – 2)(x – 1) > 0
⇒ (x –2)(x – 1) > 0 ⇒ x – 2 > 0, x – 1 > 0,
or x – 2 < 0, x – 1 < 0
⇒ x > 2 or x < 1 ⇒ x ∈ (2, ∞) or x ∈(– ∞, 1)
∴ f is increasing in (– ∞, 1] U [2, ∞)
(b) For f to be decreasing,

Question. Find the intervals in which the following function is (a) increasing (b) decreasing
f (x) = x³ – 12x² + 36x + 17
Answer. Here, f(x) = x3 – 12x² + 36x + 17
⇒ f’ (x) = 3x² – 24x + 36 = 3(x² – 8x + 12)
⇒ f’ (x) = 3(x – 2)(x – 6)
So, critical points are x = 2 and x = 6.
Required disjoint intervals are (–∞, 2), (2, 6) and (6, ∞).

Hence, f(x) is increasing in (– ∞ 2] U [6, ∞) and decreasing in [2, 6].

Question. Find the intervals in which the function f (x) = (x – 1) (x – 2)² is.
(a) increasing
(b) decreasing
Answer. We have, f(x) = (x – 1) (x – 2)²
⇒ f ‘(x) = 2 (x – 1) (x – 2) + (x –2)²
= (x – 2) (2x – 2 + x – 2)
⇒ f ‘(x) = (x – 2) (3x – 4)
For critical points, f ‘(x) = 0

Question. Find the equation of the tangent to the curve y = x⁴ – 6x³ + 13x² – 10x + 5 at the point x = 1.
Answer. Here, y = x⁴ – 6x³ + 13x² – 10x + 5                      …(i)
When x = 1, y = 1 – 6 + 13 – 10 + 5 = 3.
We want to find tangent to (i) at P(1, 3).
Differentiating (i) w.r.t. x, we get

Hence the equation of the tangent to (i) at P(1, 3) is y – 3 = 2(x – 1) ⇒ y = 2x + 1

Question. Find the equation of the tangent to the curve 4x² + 9y² = 36 at the point (3 cosθ, 2 sinθ).
Answer. The given curve is
4x² + 9y² = 36                             …(i)

Question. Find the equations of tangents to the curve y = (x² – 1)(x – 2) at the points where the curve cuts the x-axis.
Answer. The given curve is
y = (x² – 1)(x – 2)                                   …(i)
It meet x – axis, where y = 0
∴ (x² – 1)(x – 2) = 0 ⇒ x = 1, –1, 2
∴ We want to find tangents at (1, 0)(–1, 0) and (2, 0)
Now from equation (i), we get
y = x³ – 2x² – x + 2

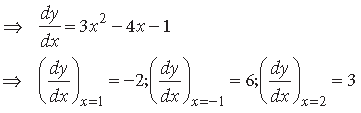
Now, tangent at (1, 0) : y – 0 = –2(x – 1) ⇒ 2x + y = 2
tangent at (–1, 0) : y – 0 = 6(x + 1) ⇒ y = 6x + 6
tangent at (2, 0) : y – 0 = 3(x – 2) ⇒ y = 3x – 6

Question. Find the equation of tangent to the curve x = sin 3t, y = cos2t at t = π/4.
Answer. The curve is x = sin 3t; y = cos 2t

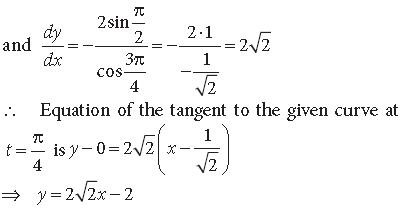
Question. Find the points on the curve y = x³ at which the slope of the tangent is equal to y-coordinate of the point.
Answer. We have, y = x³              …(i)
Differentiating (i), w.r.t. x, we get

Since it is given that slope of tangent is equal to the y-coordinate of the point.

⇒ 3x² = x³                         [using (i)]
⇒ x²(3 – x) = 0
⇒ x = 0 or x = 3
When x = 0, then from (i), y = 0
When x = 3, then from (i), y = 33 = 27
∴ The required points are (0, 0) and (3, 27).

Question. Find the equation of the tangent to the curve

at the point, where it cuts the x-axis.
Answer. The equation of curve is

This cuts the x-axis at the point where y = 0.
Putting y = 0 in the equation, we get x = 7.
Thus the point of contact is (7, 0).

Question. Find the equation of tangent to the curve x = sin 3t, y = cos3t, at t = π/4
Answer. We have, x = sin3t and y = cos3t

Question. At what points will the tangent to the curve y = 2x³ – 15x² + 36x – 21 be parallel to x-axis.
Also, find the equations of tangents to the curve at those points.
Answer. The given curve is y = 2x³ – 15x² + 36x – 21.

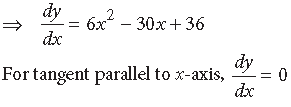
∴ 6x² – 30x + 36 = 0
⇒ 6(x² – 5x + 6) = 0 ⇒ 6(x – 3) (x – 2) = 0
⇒ x = 3, x = 2
When x = 3, y = 6 and when x = 2, y = 7
∴ The points are (3, 6) and (2, 7)
Equation of tangent at (3, 6) is,
(y – 6) = 0 (x – 3) ⇒ y = 6
Similarly, equation of tangent at (2, 7) is,
y – 7 = 0 ⇒ y = 7

Question. Find the approximate value of f (3.02), upto 2 places of decimals, where f(x) =3x²+ 5x + 3.
Answer. Given f(x) = 3x² + 5x + 3 ⇒ f ‘(x) = 6x + 5
… f(x + Δx) ≈ f(x) + Δxf'(x), where Δx → 0
Replace x = 3 and Δx = 0.02 in above relation
∴ f(3 + 0.2) ≈ f(3) + ( 0.0 2)f ‘(3)
⇒ f(3 .02) ≈ [3(3)² + 5(3) + 3] + (0.02)[6(3) + 5]
⇒ f(3.02) ≈ 45.46

Question. Using differentials, find the approximate value of (3.968)3/2.
Answer.

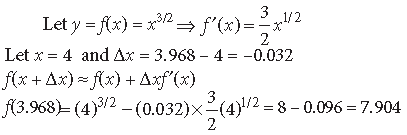
Question. Using differentials, find the approximate value of √49.5 .
Answer.

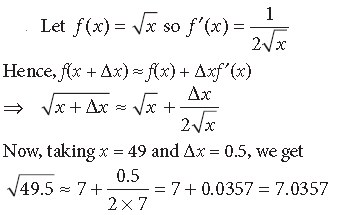
Question. If the radius of a sphere is measured as 9 cm with an error of 0.03 cm, then find the approximate error in calculating its surface area.
Answer. Let r be the radius of sphere and Δr be the error in measuring the radius. then, r = 9 cm, Δr = 0.03 cm Now surface area S of the sphere is S = 4πr²

This is the approximate error in calculating surface area.

Question. If f(x) = 3x² + 15x + 5, then find the approximate value of f(3.02), using differentials.
Answer. Given, f (x) = 3x² + 15x + 5
⇒ f ‘(x) = 6x + 15
Also, f (x + Δx) ≈ f (x) + Δx f ‘(x),
∴ f (x + Δx) ≈ 3x² + 15x + 5 + Δx(6x + 15)
Taking x = 3 and Δx = 0.02, we get
f(3.02) ≈ 3 × 32 + 15 × 3 + 5 + 0.02 (6 × 3 + 15) = 77 + 0.66
⇒ f(3.02) ≈ 77.66

Long Answer Type Questions

Question. Find the intervals in which f(x) = sin 3x – cos 3x, 0 < x < π, is strictly increasing or strictly decreasing. 
Answer. f(x) = sin 3x – cos 3x ⇒ f’ (x) = 3 cos 3x + 3 sin 3x
f’ (x)= 0 ⇒ 3 cos 3x = –3 sin 3x
⇒ cos 3x = – sin 3x ⇒ tan 3x = –1

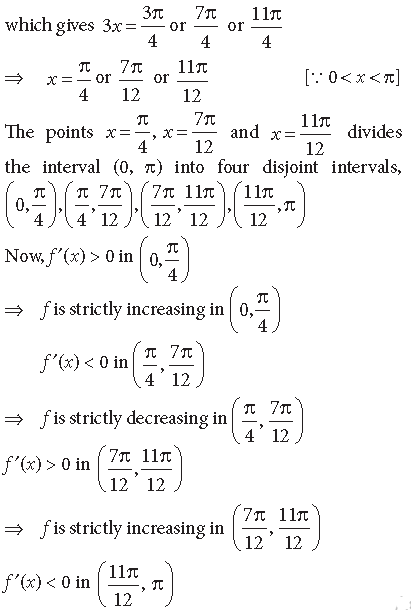
Question. Prove that the function f defined by f(x) = x² – x + 1 is neither increasing nor decreasing in (–1, 1). Hence, find the intervals in which f(x) is (i) strictly increasing (ii) strictly decreasing. 
Answer.

Here, f(x) = x2 – x + 1; x 􀂏(–1, 1)
⇒ f’ (x) = 2x – 1

Question. Find the intervals in which the function f given by f(x) = sinx – cosx, 0 ≤ x ≤ 2π is strictly increasing or strictly decreasing. 
Answer. We have f(x) = sin x – cos x, 0 ≤ x ≤ 2π
f’ (x) = cos x + sin x
For critical points f’ (x) = 0
∴ cos x + sin x = 0
⇒ sin x = – cos x ⇒ tan x = – 1

Question. Find the intervals in which the following function f(x) = 20 – 9x + 6x² – x³ is
(a) strictly increasing (b) strictly decreasing
Answer. Here, f(x) = 20 – 9x + 6x² – x³
⇒ f'(x) = – 9 + 12x – 3x² = – 3[3 – 4x + x²]
⇒ f ‘(x) = –3(x – 3)(x – 1)
So, critical points are x = 1 and x = 3.
Required disjoint intervals are (–∞, 1), (1, 3) and (3, ∞).

(a) f(x) is strictly increasing in (1, 3)
(b) f(x) is strictly decreasing in (– ∞, 1) U(3, ∞)

Question. Find the equation of tangents to the curve y = cos(x + y), –2π ≤ x ≤ 2 π that are parallel to the line x + 2y = 0.
Answer. Let the point of contact of one of the tangents be (x₁, y₁).
Then (x₁, y₁) lies on y = cos (x + y)
∴ y₁ = cos(x₁, + y₁)                         …(i)
Since the tangents are parallel to the line
x + 2y = 0. Therefore,
Slope of tangent at (x₁, y₁) = slope of line x + 2y = 0

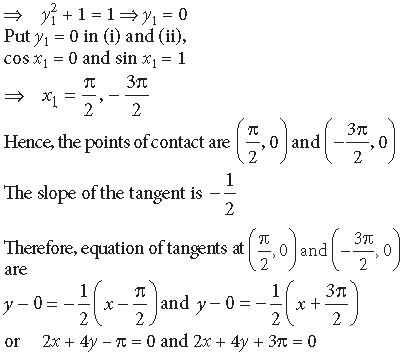
Question. Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is 4r/3. Also find maximum volume in terms of volume of the sphere
Answer.

Question. Prove that the least perimeter of an isosceles triangle in which a circle of radius r can be inscribed is 6 3r.
Answer. Let ΔABC be the given triangle and AD is the altitude of the isosceles triangle ABC.

⇒ –2(1 – sin²x) + 4 sin³ x + 4 sin²x = 0
⇒ 2sin³x + 3sin²x – 1 = 0
⇒ (sinx + 1) (2sin²x + sinx – 1) = 0
sinx cannot be –1 because ‘x’ cannot be more than 90°.
So, 2 sin²x + sinx – 1 = 0
⇒ (2sinx –1) (sinx + 1) = 0
Again sinx cannot be –1.

Question. If the sum of lengths of hypotenuse and a side of a right angled triangle is given, show that area of triangle is maximum, when the angle between them is π/3
Answer. Let ABC be a right angled triangle with BC = x,
AC = y such that x + y = k, where k is any constant.
Let θ be the angle between the base and the hypotenuse.
Let P be the area of the triangle.

Question. The sum of the surface areas of a cuboid with sides x, 2x and x/3 and a sphere is given to be constant. Prove that the sum of their volumes is minimum, if x is equal to three times the radius of sphere. Also find the minimum value of the sum of their volumes. 
Answer. Surface area of cuboid = 2(lb + bh + hl)

Question. Find the value of p for which the curves x² = 9p(9 – y) and x² = p(y + 1) cut each other at right angles.
Answer. The given curves are
x² = 9p(9 – y) … (i)
x² = p(y + 1) … (ii)
Clearly p ≠(as p = 0 ⇒ both curves become same)
Solving (i) and (ii), we get
9p(9 – y) = p(y + 1)

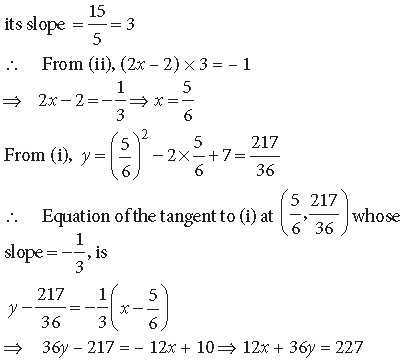
Question. Find the equation of the tangent line to the curve y = x² – 2x + 7 which is (i) parallel to the line 2x – y + 9 = 0, (ii) perpendicular to the line 5y– 15x = 13.
Answer. The given curve is y = x² – 2x + 7              …(i)

= Slope of the tangent to (i) at (x, y)                       ..(ii)
(i) The tangent is parallel to the line 2x – y + 9 = 0
Its slope = 2
∴ From (ii), 2x – 2 = 2 ⇒ x = 2
From (i), y = 22 – 2 × 2 + 7 = 7
∴ Equation of the tangent to (i) at (2, 7) whose slope = 2, is
y – 7 = 2(x – 2) ⇒ 2x –y + 3 = 0
(ii) The tangent is perpendicular to the line
5y – 15x = 13,

Question. Find the equation of the normal at a point on the curve x² = 4y which passes through the point (1, 2). Also find the equation of the corresponding tangent.
Answer. We have, x² = 4y
Differentiating w.r.t. x, we get

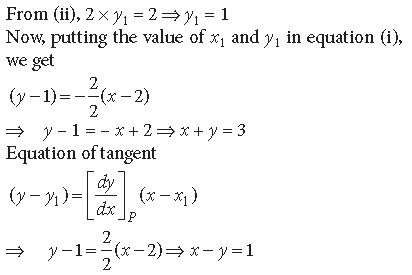
Question. Find the equations of tangents to the curve 3x² – y² = 8, which pass through the point
Answer. We have, 3×2 – y2 = 8              …(i)
Differentiating w.r.t. x, we get

Question. For the curve y = 4x³ – 2x⁵, find all the points on the curve at which the tangent passes through the origin. 
Answer. The given curve is
y = 4x^{3 –} 2x⁵

Question. Find the maximum area of an isosceles triangle inscribed in the ellipse

with its vertex at one end of the major axis.
Answer.

From P, draw a line parallel to y-axis and produce it to meet the ellipse at Q, then PRQ is an isosceles triangle. Let A be its area, then

Question. An open tank with a square base and vertical sides is to be constructed from a metal sheet so as to hold a given quantity of water. Show that the cost of the material will be least when the depth of the tank is half of its width.
Answer. Let x be the side of square base and y be the height of the open tank.
∴ l = x, b = x and h = y
where l, b and h be the length, breadth and height of tank respectively.

The cost of the material will be least if the total surface area is least.
Total surface area of tank (S) = x² + 4xy

Question. A wire of length 28 cm is to be cut into two pieces. One of the two pieces is to be made into a square and the other into a circle. What should be the length of the two pieces so that the combined area of these is minimum ?
Answer. Let the length of the piece bent into the shape of a circle be x m and length of the other piece bent into the shape of a square is (28 – x) m.
Circumference of circle = 2πr
⇒ 2πr = x ⇒ r = x/2π
⇒ Area of the circle = π(radius)²

Question. Find the equation of the tangent to the curve

which is parallel to the line 4x – 2y + 5 = 0
Answer.

Question. Prove that the curve x = y² and xy = k cut at the right angles if 8k² = 1.
Answer. The given curves are
y2 = x            … (i)
xy = k             …(ii)
Differentiating (i) w.r.t. x, we get

Question. Prove that all normals to the curve x = a cos t + at sin t, y = a sint – at cos t are at a constant distance ‘a’ from the origin.
Answer. Here, x = a cos t + at sin t
y = a sin t – at cos t